home \ courses \ music theory II \ page 6

Adding intervals

Before we look at intervals tha go down in pitch instead of going up, we'll take a look at how intervals can be put together to from an octave.

It is fairly simple. You want to find to intervals that together make an octave. The following list shows which intervals can be added:

• A major second + a minor seventh = one perfect octave. Eg. C-D + D-C = C-C'
• A major third + a minor sixth = one perfect octave. E.g. C-E + E-C = C-C'
• A perfect fourth + a perfect fifth = one perfect octave. E.g. C-F + F-C = C-C'
• A perfect fifth + a perfect fourth = one perfect octave. E.g. C-G + G-C = C-C'
• A major sixth + a minor third = one perfect octave. E.g. C-A + A-C = C-C'
• A major seventh + a minor second = one perfect octave. Eg. C-B + B-C = C-C'

As you may notice, the type of intervals changes form major to minor, or stays perfect.

Also notice: e.g. a major third + a minor sixth is one octave, and aslo a minor third + a major sixth is one octave. If one is major, than the other is minor.

The same is with diminished and augmented:
An augmented fourth + a diminidhed fifth is an octave, jus as a dimished fourth + an augmented fifth is an octave.

Now this all is very theoretically. Let's see how we can actually use this information.

Negative intervals

Negative intervals go donw in pitch. E.g. Instead of going up a third, you can go down a third:

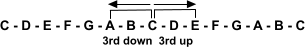
When you go down a minor third starting at C, the second tone is the A. If you would go the other way from C to A, You would have a major sixth:

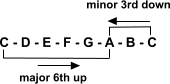
This way it can be easy to go down an interval. Another example: let's go a minor seventh down. We already now that a seventh + a second = an octave. We also now that a minor seventh + a major second = an octave.
So, if we go down a minor seventh, starting at C, that would be the same as first hoing an octave down, after that going a major second up. A major second on C is a D:

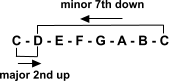
There is a lot of theory, and math, in intervals. Once you start analyzing them, the pieces will eventually fall into place. There is one thing left in this part of the course: the relation between scales and intervals.

<<< Previous page   |   Next page >>>